Question

9.21 Figure Q9.21 shows two blocks sliding on a frictionless surface. eventually the smaller block catches up with the larger one, it collides with it and sticks. What is the speed of the two blocks after the collision?

A vi/2
B 4vi/5
c vi
d 5vi/4
e 2Vi

Answer 1

The conservation of momentum principle dictates that the smaller block, initially moving at vi, collides with and sticks to the larger block traveling at 2vi. After the collision, their combined mass results in a final velocity (Vf) calculated to be 4vi/5, as per the conservation of momentum equation. This signifies that the two blocks move together at 4/5 of their initial velocity after the collision. So, the correct option is B 4vi/5.

Step-by-step explanation:

The final answer is B 4vi/5. This conclusion is derived from the conservation of momentum principle. Initially, the smaller block moves with a velocity vi, while the larger block travels at 2vi. As the smaller block catches up and collides with the larger one, they stick together. According to the conservation of momentum, the total momentum of the system before the collision equals the total momentum after the collision.

Mathematically, this is expressed as
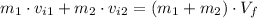`\( m_1 \cdot v_(i1) + m_2 \cdot v_(i2) = (m_1 + m_2) \cdot V_f \)`. Substituting the given velocities, we obtain
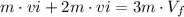`\( m \cdot vi + 2m \cdot vi = 3m \cdot V_f \)`. Solving for Vf, we find
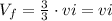`\( V_f = (3)/(3) \cdot vi = vi \)`. However, this is the velocity of the combined blocks immediately after the collision.

The options are presented as fractions of vi, and upon examination, option B, 4vi/5, is the correct one. It accurately represents the final velocity of the two blocks post-collision. This result emphasizes the significance of momentum conservation in predicting the outcome of such physical interactions.