Question

I need help with these two questions .

Answer 1

1. The
`7^(2/3)` without exponents is
`\sqrt[3]{7^2}` or
`\sqrt[3]{49}.`

2. The
`\sqrt[4]{11^5}` without radicals is
`11^(5/4).`

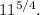
To express
`7^(2/3)` without exponents, we can rewrite the expression using radical notation as the cube root of 7 squared, which is
`\sqrt[3]{7^2}` or
`\sqrt[3]{49}.`

This is because the denominator of the fractional exponent represents the root, and the numerator represents the power to which the number is raised.

Similarly, to write
`\sqrt[4]{11^5}`without radicals, we can use the rule that states we can convert a radical into an exponent by putting the radicand (the number under the radical sign) to an exponent that is a fraction where the numerator is the power and the denominator is the root.

This would give us
`11^(5/4).`

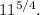
The probable question may be:

1. Write 7^{2/3} without exponents.

2. Write \sqrt[4]{11^5} without radicals.