Question

The circle below has center O, and its radius is 4 ft. Given that m ZAOB=50°, find the area of the shaded region and the length of the arc ADB.

Give exact answers in terms of , and be sure to include the correct units in your answer.

Area of shaded region:

Length of ADB:

Answer 1

Area of the shaded region: 1.31π - 5.84
`ft ^2`

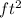
Length of arc ADB: 4π/7 ft

Area of the shaded region:

Sector AOB: The area of sector AOB can be calculated using the formula: Area of sector = (θ/360) * πr², where θ is the central angle in degrees, r is the radius, and π is the constant pi (approximately 3.14159). In this case, θ = 50° and r = 4 ft. Therefore, the area of sector AOB = (50/360) * π * 4² = (5/36) * 16π = 10π/9
`ft ^2`.

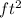Triangle AOB: The area of triangle AOB can be calculated using the formula: Area of triangle = 1/2 * base * height. Since AO and BO are radii, they are equal in length (4 ft) and form the base of the triangle. The height of the triangle can be found using the sine function: sin(θ/2) = height / base, where θ is the central angle. Solving for the height, we get: height = sin(50°/2) * 4 ≈ 2.92 ft. Therefore, the area of triangle AOB = 1/2 * 4 * 2.92 ≈ 5.84
`ft ^2`.

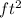Shaded region: The shaded region is the difference between the area of sector AOB and the area of triangle AOB. Therefore, the area of the shaded region = 10π/9
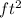`ft ^2`- 5.84
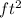`ft ^2`≈ 1.31π - 5.84
`ft ^2`.

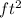Length of arc ADB:

Central angle: Arc ADB is half of the central angle AOB because AB is a diameter of the circle. Therefore, the central angle of arc ADB is θ/2 = 50°/2 = 25°.

Arc length: The length of an arc can be calculated using the formula: Arc length = (θ/360) * 2πr. Substituting the values for θ and r, we get the length of arc ADB = (25/360) * 2π * 4 = (1/14) * 16π = 4π/7 ft.