Question

find ln(12) rounded to the nearest tenth. if necessary, include a leading 0 with any decimals less than 1. for example, 0.5 instead of .5.

Answer 1

ln(1/2) rounded to the nearest tenth is roughly negative zero point seven, or -0.69.

Let's find
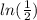`\(ln((1)/(2))\)` rounded to the nearest tenth.

We can find the value of the expression using a calculator or by using the following steps:

1. Use the logarithm rule:
`$\ln(a^b)`=
`b\ln(a)$.`

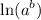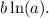
2. Simplify the expression:
`$\ln((1)/(2))` =
`\ln(2^(-1))` =
`-1\ln(2)$.`

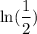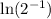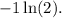
3. Calculate the value of
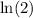`$\ln(2)$` using a calculator.

4. Round the answer to the nearest tenth.

Steps to solve:

1. Apply the logarithm rule:

`$$\ln((1)/(2)) = \ln(2^(-1))$$`

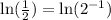
2. Simplify the expression:

`$$=-1\ln(2)$$`

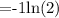
3. Calculate the value of ln(2) using a calculator:

`$$\ln(2) \approx 0.69314718056$$`

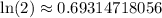
4. Round the answer to the nearest tenth:

`$$-1\ln(2) \approx -0.69$$`

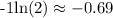
Answer:

`$$\ln((1)/(2)) \approx -0.69$$`

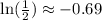
Question: