Question

An instructor gives the same final exam every year. She knows that the y = 78 but the

population variance is unknown. She thinks that her current class is advanced, and will do
better than past classes (have a higher mean). The class consists of 25 students, and their final
exam class mean was 86 with a sample standard'deviation of 10. Did her class do statistically
better than previous classes? Conduct an appropriate statistical test using a = .05. Write your
answer using the 8 steps of hypothesis testing

Answer 1

The evidence suggests that the current class performed statistically better than previous classes on the final exam at a significance level of 0.05.

Let's conduct hypothesis testing to determine if the current class performed statistically better than the previous classes on the final exam. We'll use an appropriate statistical test (in this case, a one-sample t-test) and follow the 8 steps of hypothesis testing:

8 Steps of Hypothesis Testing:

State the Hypotheses

Null Hypothesis (H0): The current class did not perform better than previous classes. μ = 78

Alternative Hypothesis (H1): The current class performed better than previous classes. μ > 78

Choose the Significance Level

The significance level (α) is given as 0.05.

Select the Test Statistic

Since the population variance is unknown and the sample size is relatively small (n = 25), we'll use a t-test for a single sample.

Formulate the Decision Rule

With a significance level of 0.05 and a one-tailed test (since we're checking if the mean is greater), we'll find the critical t-value using a t-table or a statistical software.

Compute the Test Statistic

The formula for the t-test for a single sample is:

t=
`(x- \mu)/((s)/(√(n) ) )`

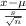
Where:

x is the sample mean (86)

μ is the population mean (78)

s is the sample standard deviation (10)

n is the sample size (25)

Make a Decision

Calculate the t-statistic and compare it to the critical t-value obtained in

Make a Decision

If the calculated t-value falls into the rejection region (greater than the critical t-value), we reject the null hypothesis.

Conclusion

Let's calculate the t-statistic and make a decision:

t= 86−78/ 10/25

​t= 8/2 =4

Using a t-table or statistical software with 24 degrees of freedom (n - 1), the critical t-value at α = 0.05 (one-tailed test) is approximately 1.711.

Since the calculated t-value (4) is greater than the critical t-value (1.711), we reject the null hypothesis.

Conclusion:

The evidence suggests that the current class performed statistically better than previous classes on the final exam at a significance level of 0.05.