Question

Analysis shows 2.123 x 10^21 molecules of X2 are present in 0.250 g of X2.

What is the molar mass of X2?
[?] X2

Answer 1

The molar mass of
`\(X_2\)` is approximately
`\(7.08 \, \text{g/mol}\)`.

To determine the molar mass
`(\(M\))` of a molecule
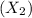`(\(X_2\))`, you can use the formula:

`\[ M = \frac{\text{Mass (g)}}{\text{Amount of substance (moles)}} \]`

In this case, you're given the amount of substance in molecules
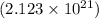`(\(2.123 * 10^(21)\))` and the mass of
`\(X_2\)` in grams (0.250 g).

Step 1: Determine the number of moles
`(\(n\))` using Avogadro's number:

`\[ \text{Amount of substance (moles)} = \frac{\text{Number of molecules}}{\text{Avogadro's number}} \\\[ n = (2.123 * 10^(21))/(6.022 * 10^(23)) \]`

Step 2: Calculate the molar mass
`(\(M\))`:

`\[ M = \frac{\text{Mass (g)}}{\text{Amount of substance (moles)}} \]`

`\[ M = \frac{0.250 \, \text{g}}{n} \]`

Plug in the value of n from step 1.

`\[ M = \frac{0.250 \, \text{g}}{(2.123 * 10^(21))/(6.022 * 10^(23))} \]`

Calculate the result.

The unit for molar mass will be grams per mole (g/mol), which is the standard unit for expressing molar mass.

Performing the calculation:

`\[ n = (2.123 * 10^(21))/(6.022 * 10^(23)) \approx 0.0353 \, \text{mol} \]`

`\[ M = \frac{0.250 \, \text{g}}{0.0353 \, \text{mol}} \approx 7.08 \, \text{g/mol} \]`

So, the molar mass of
`\(X_2\)` is approximately
`\(7.08 \, \text{g/mol}\)`.