Answer:
Area = 35.7x in²
Explanation:
The formula for the area of a trapezoid is:

In this case:
To find the area of the trapezoid, we can substitute the given values into the formula and solve for A:

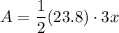
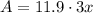
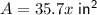
Therefore, the area of the trapezoid is 35.7x in².
Please note that as the height (h) of the trapezoid in the given diagram is 3x in, we can only find the area in terms of x. If you have been given the value of x, you can substitute this into 35.7x to find the area of the trapezoid.