Question

Wavelength of light that has an energy of 1.50x10^-18 J ??

Answer 1

The wavelength of light with an energy of
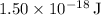`\(1.50 * 10^(-18) \, \text{J}\)` is approximately
`\(1.32 * 10^(-7) \, \text{m}\)`.

To find the wavelength
`(\(\lambda\))` of light given its energy
`(\(E\))`, you can use the following formula:

`\[ E = h \cdot \\u \]`

Since
`\(E\)` can also be expressed as
`\(hc/\lambda\)` (where
`\(h\)` is Planck's constant and
`\(c\)` is the speed of light), we can set up the equation:

`\[ hc/\lambda = E \]`

Solving for
`\(\lambda\)`:

`\[ \lambda = (hc)/(E) \]`

Given
`\(E = 1.50 * 10^(-18) \, \text{J}\)`,
`\(h = 6.626 * 10^(-34) \, \text{J} \cdot \text{s}\)`, and
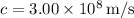`\(c = 3.00 * 10^8 \, \text{m/s}\)`, you can substitute these values into the equation:

`\[ \lambda = \frac{(6.626 * 10^(-34) \, \text{J} \cdot \text{s}) \cdot (3.00 * 10^8 \, \text{m/s})}{1.50 * 10^(-18) \, \text{J}} \]`

Calculate this expression to find the wavelength
`\(\lambda\)`. The unit of wavelength will be meters.

`\[ \lambda \approx (1.987 * 10^(-25))/(1.50 * 10^(-18)) \, \text{m} \]`

`\[ \lambda \approx 1.32 * 10^(-7) \, \text{m} \]`

So, the wavelength of light with an energy of
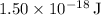`\(1.50 * 10^(-18) \, \text{J}\)` is approximately
`\(1.32 * 10^(-7) \, \text{m}\)`.

The probable question may be:

"What is the wavelength of light that has an energy of 1.50x
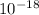`10^{-18` J "