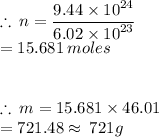Question

What is the mass of 9.44 × 102 ⁴ molecules of NO2? The molar mass of NO₂ is 46.01 g/mol.

-294 g
-721 g
-341 g
-205 g
-685 g

Answer 1

`\huge{ \boxed{721 \: g}}`

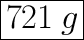
Step-by-step explanation:

The mass of the
`NO_2` molecules can be found by using the formula:

m = n × M

where:

m is the mass in grams

M is the molar mass in g/mol

n is the number of moles

From the question:

M = 46.01 g/mol

From the question, the number of moles was not given, however the number of molecules was given. So the number of molecules is then used to find the number of moles by using the formula:

`n = (N)/(L)`

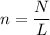
where:

n is the number of moles

N is the number of entities

L is the Avogadro's constant which is

6.02 × 10²³ entities

From the question:

N = 9.44 × 10²⁴ molecules

`\therefore \: n = \frac{9.44 * {10}^(24) }{6.02 * {10}^(23) } \\ = 15.681 \: moles \\ \\ \\ \therefore \: m = 15.681 * 46.01 \\ = 721.48 \approx \:721g`

Answer 2

To find the mass of 9.44 x 10²⁴ molecules of NO₂, convert molecules to moles using Avogadro's number, then multiply by the molar mass of NO₂. The answer is approximately 721 grams.

Step-by-step explanation:

The question asked is about determining the mass of a certain number of molecules of nitrogen dioxide (NO2).

To find the mass of 9.44 × 1024 molecules of NO2, we first need to convert the number of molecules to moles using Avogadro's number, which is 6.022 × 1023 molecules per mole:

1. Calculate the number of moles: Number of moles = [Number of molecules] / [Avogadro's number] = [9.44 × 1024] / [6.022 × 1023 mol-1] = 15.67 moles.
2. Use the molar mass of NO2 to get the mass: Mass = [Number of moles] × [Molar mass of NO2] = [15.67 moles] × [46.01 g/mol] = 720.78 g.

This calculation shows that the correct mass for 9.44 × 1024 molecules of NO2 is approximately 721 grams.