To prove the identity
 , let's follow a step-by-step proof using the given rules
, let's follow a step-by-step proof using the given rules
Identity to be Proven:
![\[ (\sin(\pi - x))/(\sin(x + (\pi)/(2))) = \tan x \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/u27wjnukynws58ri8krvyzbmc3kmlsk7ai.png)
Proof:
Step 1: We apply the Sum and Difference identity for sine:
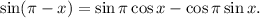
- Rule Used: Sum and Difference
![\[ (\sin(\pi - x))/(\sin(x + (\pi)/(2))) = (\sin \pi \cos x - \cos \pi \sin x)/(\sin(x + (\pi)/(2))) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/olhj7xkt858kgrwy390wrzco401ftg4zr4.png)
Step 2: We simplify the expression using the trigonometric values for
 and
and
 :
:
- Rule Used: Evaluation
![\[ (\sin(\pi - x))/(\sin(x + (\pi)/(2))) = (0 \cdot \cos x - (-1) \cdot \sin x)/(\sin(x + (\pi)/(2))) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/3q8k1w8pytyvzva8bn3oo8hybraqcm5fbz.png)
![\[ = (\sin x)/(\sin(x + (\pi)/(2))) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/aculpnvp1xq5tw8lsnf3xpt7a8v21ufnaj.png)
Step 3: We apply the Sum and Difference identity for sine:
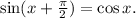
- Rule Used: Sum and Difference
![\[ (\sin(\pi - x))/(\sin(x + (\pi)/(2))) = (\sin x)/(\cos x) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/rpfvi1eocbckiasl9i99zwfwbd9g4fig27.png)
Step 4: We use the Quotient identity for tangent:
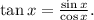
- Rule Used: Quotient
![\[ (\sin(\pi - x))/(\sin(x + (\pi)/(2))) = \tan x \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/u27wjnukynws58ri8krvyzbmc3kmlsk7ai.png)
The proof is complete, and the given identity has been verified using the provided rules.