Step-wise proof:
1.
 .
.
2.
 (Given).
(Given).
3.
 is common to both triangles
is common to both triangles
 and
and
 (Reflexive Property of Congruence).
(Reflexive Property of Congruence).
4. Triangles
 and
and
 are congruent by ASA (Angle-Side-Angle).
are congruent by ASA (Angle-Side-Angle).
5. By CPCTC (Corresponding Parts of Congruent Triangles are Congruent),
 .
.
Therefore, option f is correct
To prove that
 , we need to use the information given and find a congruence postulate or theorem that justifies the segments are congruent.
, we need to use the information given and find a congruence postulate or theorem that justifies the segments are congruent.
Given:
-
 is an angle bisector of
is an angle bisector of
 , which means
, which means
 .
.
-
 .
.
To prove:
-
 .
.
Let's look at the potential congruences to see which one leads to the proof:
a)
 ;
;
 ;
;
 is common
is common
 by Angle-Angle-Side.
by Angle-Angle-Side.
This option cannot be correct immediately because
 and
and
 are not necessarily congruent by the given information.
are not necessarily congruent by the given information.
b)
 ;
;
 ;
;
 is common
is common
 by Angle-Angle-Side.
by Angle-Angle-Side.
This option is not valid because it incorrectly identifies triangles and the angle relationships do not arise from the given information.
c)
 ;
;
 ;
;
 is common
is common
 by Angle-Angle-Side.
by Angle-Angle-Side.
This option is incorrect because it talks about congruence between angles instead of segments.
d)
 ;
;
 by Angle-Angle-Side.
by Angle-Angle-Side.
This option is not valid for the same reason as option b, it incorrectly identifies triangles and does not use the given information properly.
e)
 ;
;
 ;
;
 is common
is common
 by Angle-Angle-Side.
by Angle-Angle-Side.
This option is true due to the Isosceles Triangle Theorem (since
 , the base angles
, the base angles
 and are congruent), but it does not directly prove that
and are congruent), but it does not directly prove that
 .
.
f)
 ;
;
 ;
;
 is common
is common
 by Angle-Angle-Side.
by Angle-Angle-Side.
This option is the correct reasoning. Since
 bisects
bisects
 , the angles
, the angles
 and
and
 are congruent. We are also given that
are congruent. We are also given that
 , and by the Reflexive Property,
, and by the Reflexive Property,
 . Therefore, by Angle-Side-Angle (ASA) congruence postulate,
. Therefore, by Angle-Side-Angle (ASA) congruence postulate,
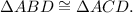
Step-wise proof:
1.
 .
.
2.
 (Given).
(Given).
3.
 is common to both triangles
is common to both triangles
 and
and
 (Reflexive Property of Congruence).
(Reflexive Property of Congruence).
4. Triangles
 and
and
 are congruent by ASA (Angle-Side-Angle).
are congruent by ASA (Angle-Side-Angle).
5. By CPCTC (Corresponding Parts of Congruent Triangles are Congruent),
 .
.
Therefore, the correct statement that describes the solution is:
f)
 ;
;
 ;
;
 is common
is common
 by Angle-Angle-Side.
by Angle-Angle-Side.