Question

The function (y = 2 cos left(3left(x+ 25/3right)right) + 1) has a phase shift (or horizontal shift) of

A) 3
B) 2
C) -2/3
D) 1

Answer 1

C) -2/3

Step-by-step explanation:

The given function is
`\(y = 2 \cos \left(3\left(x + (25)/(3)\right)\right) + 1\)`. The general form of the cosine function is
`\(y = A \cos(B(x - C)) + D\)`, where A is the amplitude, B is the frequency (or period), C is the phase shift, and D is the vertical shift.

In this case, the coefficient of
`\(x\)` inside the cosine function is 3, indicating that the frequency is affected. The standard form of the cosine function is
`\(y = \cos(x)\)`, and any modification inside the function affects the phase shift. The formula for the phase shift (C) is
`\(C = (C_1)/(B)\)` , where
`\(C_1\)` is the value inside the parentheses.

For the given function,
`\(C_1 = (25)/(3)\) and \(B = 3\).` Plugging these values into the formula, we get
`\(C = ((25)/(3))/(3) = -(2)/(3)\)`. Therefore, the correct answer is C) -2/3, indicating that the function has a horizontal shift to the left by
`\(-(2)/(3)\).`

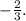
Understanding the phase shift is crucial for graphing and analyzing periodic functions like cosine, as it helps determine the position of the graph along the x-axis. In this case, the phase shift of
`\(-(2)/(3)\)` signifies that the graph is shifted to the left by
`\(-(2)/(3)\)` units compared to the standard cosine function.