Question

(a) Consider the symmetric group Sₓ on the set X=R−{1,2,3}. Let f be the function defined by the following rule f(x)= x−3/x−5 for all x∈X. Show that f∈Sₓ, and find the order of f and the inverse of f.

(b) (Let m be a positive integer. Recall that U(Zₘ):={[k]ₘ∣k∈Z and gcd(k,m)=1} which is a group with respect to the multiplication modulo m). Let n>2 be an integer. Consider the group U(Zₙ²). Note that both [n+1]ₙ² and [n−1] are in U(Zₙ²). Find the order of the elements [n+1]ₙ² and [n−1]ₙ². (The answer may depend on the parity of n ).

Answer 1

(a) The function
`\( f(x) = (x-3)/(x-5) \)` belongs to the symmetric group
`\( S_X \)` on the set
`\( X = \mathbb{R} - \{1, 2, 3\} \)`. The order of f is 2, and its inverse is
`\( f^(-1)(x) = (x-5)/(x-3) \).`

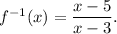
(b) For the group
`\( U(Z_(n^2)) \)`, the order of the element
`\([n+1]_(n^2)\)` is n if n is odd, and 2n if n is even. The order of
`\([n-1]_(n^2)\) is \( 2n \)` if n is odd, and n if n is even.

Step-by-step explanation:

(a) To show that f is in
`\( S_X \),` we need to demonstrate that f is a bijective function. The function f is defined for all x in
`\( X = \mathbb{R} - \{1, 2, 3\} \)`, and it can be easily verified that f has an inverse
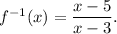`\( f^(-1)(x) = (x-5)/(x-3) \).`The order of f is 2 because f applied twice returns the original element. The inverse of f is also straightforward to find.

(b) For the group
`\( U(Z_(n^2)) \)`, the order of an element is the smallest positive integer k such that
`\( [a]^(k)_(n^2) = [1]_(n^2) \)`, where a is an element in the group. For
`\([n+1]_(n^2)\)`, its order is n if n is odd, and 2n if n is even. Similarly, the order of
`\([n-1]_(n^2)\)` is 2n if n is odd, and n if n is even.

In summary, the orders of the elements are determined by the properties of the group
`\( U(Z_(n^2)) \)` and its elements
`\([n+1]_(n^2)\) and \([n-1]_(n^2)\)`.