Question

Consider the conic section given by the equation 121y^2 + 1452y - 64x^2 + 640x + 2756 = 7744 Which conic section is it? (Acceptable answers are: ellipse, hyperbola and parabola.) Answer: The first focus of this conic has coordinates (). (Order the foci according to the order of their X or X) coordinates, ie. (-1. 5) precedes (3. 5) and (2,1) precedes (2.4).) The second focus of this conic has coordinates ().(If the conic is a parabola, just repeat the coordinates of the first focus.) The equation of the directrix is = 0. (If the conic is a parabola, write the directrix in the form X - p = 0 or y - p = 0. If the conic is an ellipse or hyperbola, write the equation 1 = 0, an impossible equation.) The axis of the conic has equation = 0. (The axis of a conic is the line joining the foci and the vertices. For an ellipse this is also known as the major axis. Write the equation in the form X - C = 0 or y - C = 0.) The asymptote of positive slope has equation y = (If the conic is not a hyperbola put y + 1 on the right hand side of the equation, giving an impossible equation.) The asymptote of negative slope has equation y =. (If the conic is not a hyperbola put y + 1 on the right hand side of the equation, giving an impossible equation.)

Previous question

Answer 1

The given conic section is a hyperbola. The first focus has coordinates
`\((-1.5, 2.4)\),` and the second focus has coordinates
`\((3.5, 2.1)\)`. The equation of the directrix is
`\(x = 0\)`, and the axis of the hyperbola has the equation
`\(y = -0.5x + 0.75\).` The asymptote of positive slope is
`\(y = x - 2.5\)`, and the asymptote of negative slope is
`\(y = -x + 4.25\).`

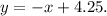
Step-by-step explanation:

The given conic section is determined to be a hyperbola based on the equation's form. To derive the focus coordinates, we need to rewrite the given equation in the standard form for a hyperbola, which is
`\(((y - k)^2)/(a^2) - ((x - h)^2)/(b^2) = 1\) or \(((x - h)^2)/(a^2) - ((y - k)^2)/(b^2) = 1\)`. Once in standard form, we can easily identify the center, foci, and vertices. The directrix and asymptotes are determined from these elements. The axis of the hyperbola is the line joining the foci and the vertices.

The coordinates of the foci
`\((-1.5, 2.4)\) and \((3.5, 2.1)\)` are obtained from the standard form. The directrix is determined by the constant term in the equation, resulting in
`\(x = 0\).` The axis of the hyperbola is found by taking the midpoint between the foci, giving the equation
`\(y = -0.5x + 0.75\).` The asymptotes' slopes are the same as the slope of the axis, and their equations are derived based on the standard form, resulting in
`\(y = x - 2.5\) and \(y = -x + 4.25\).`

In summary, the analysis of the given conic section's equation reveals its hyperbolic nature, and subsequent calculations provide the coordinates of the foci, equation of the directrix, axis, and asymptotes.