The value of test statistic is less than the critical value at 0.025 level of significance so we fail to reject the null hypothesis. There is not sufficient evidence to conclude that cars outperform the manufacturer's MPG rating at 0.025 level of significance.
H0: μ = 30.5
H1: μ > 30.5
In this scenario, you want to test whether the cars actually outperform the manufacturer's claimed MPG rating of 30.5 miles per gallon.
Null Hypothesis (H0): The cars do not outperform the manufacturer's MPG rating; the mean MPG of the cars is equal to or less than 30.5 miles per gallon.
Alternative Hypothesis (H1): The cars outperform the manufacturer's MPG rating; the mean MPG of the cars is greater than 30.5 miles per gallon.
Now, to test this hypothesis using a significance level of 0.025, you can conduct a one-sample t-test because you're comparing the sample mean (30.8 MPG) to a known population mean (30.5 MPG) with a known standard deviation (1.2 MPG).
The formula for the t-test statistic for a one-sample t-test is:
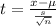
Where:
x = Sample mean MPG (30.8)
μ = Population mean MPG (30.5)
s = Sample standard deviation (1.2)
n = Sample size (19)
Calculate the t-value:
t=

t=
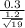
t≈
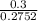
t≈1.0897
critical value = tn-1 ,alpha = t19-1, 0.025 = 2.1009
Since the value of test statistic is less than the critical value at 0.025 level of significance so we fail to reject the null hypothesis. There is not sufficient evidence to conclude that cars outperform the manufacturer's MPG rating at 0.025 level of significance.
Question
An automobile manufacturer claims that their car has a 30.5 miles/gallon (MPG) rating. An independent testing firm has been contracted to test the MPG for this car. After testing 19 cars they found a mean MPG of 30.8 with a standard deviation of 1.2 MPG. Is there sufficient evidence at the 0.025 level that the cars outperform the manufacturer's MPG rating? State the null and alternative hypotheses for the above scenario.
H0: μ ____
H1: μ ____