Answer:
(a) Annual: $67,699.45
(b) Semiannual: $68,330.65
(c) Monthly: $68,889.97
(d) Daily: $69,001.91
Step-by-step explanation:
To determine the value of a $44,000 investment at the end of 5 years with an annual interest rate of 9%, we can use the compound interest formula.
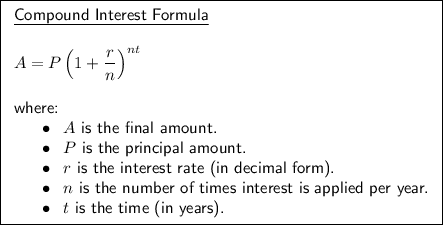
In this case:
- P = $44,000
- r = 9% = 0.09
- t = 5 years
Substitute these values into the compound interest formula:

(a) To find the value of the investment (A) when the interest is compounded annually, substitute n = 1 into the equation:




(b) To find the value of the investment (A) when the interest is compounded semi-annually, substitute n = 2 into the equation:




(c) To find the value of the investment (A) when the interest is compounded monthly, substitute n = 12 into the equation:




(d) To find the value of the investment (A) when the interest is compounded daily, substitute n = 365 into the equation:



