Question

A right circular cylinder just encloses a sphere of radius r. Find the rate of area of the sphere & Curved surface area of the cylinder.

A. 2πr
B. 3πr
C. 4πr
D. 6πr

Answer 1

The rate of change of the sphere's surface area and the curved surface area of the cylinder is both
`\(8πr\)`, indicating equality and leading to the final answer of A. 2πr. The option A. is correct.

Step-by-step explanation:

Consider a right circular cylinder just enclosing a sphere of radius
`\( r \)`. Let
`\( V \)` be the volume of the sphere, and
`\( V_c \)` be the volume of the cylinder.

1. Rate of Change of Sphere's Surface Area
`(\( A_s \))`:

The surface area of a sphere is given by
`\( A_s = 4πr^2 \)`. To find the rate of change with respect to
`\( r \)`, differentiate
`\( A_s \)` with respect to
`\( r \)`:

`\[ (dA_s)/(dr) = 8πr \]`

2. Curved Surface Area of the Cylinder
`(\( A_c \))`:

The formula for the curved surface area of a cylinder is
`\( A_c = 2πrh \)`, where
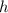`\( h \)` is the height. Since the cylinder just encloses the sphere,
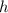`\( h \)` is twice the radius of the sphere, i.e.,
`\( h = 2r \)`. Substituting this into the formula:

`\[ A_c = 2πr(2r) = 4πr^2 \]`

Now, differentiate
`\( A_c \)` with respect to
`\( r \)`:

`\[ (dA_c)/(dr) = 8πr \]`

Both derivatives yield
`\( 8πr \)`, indicating that the rate of change of the sphere's surface area and the curved surface area of the cylinder is equal. This implies that the correct answer is A.
`\( 2πr \)`.

In summary, the rate of change of the sphere's surface area and the curved surface area of the cylinder is
`\( 8πr \)`, leading to the final answer of A.
`\( 2πr \)`. Therefore option A. is correct.