Final Answer:
The remainder when
 is divided by
is divided by
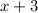 is
is
 . Option B.
. Option B.
Step-by-step explanation:
Polynomial division involves dividing the given polynomial by the divisor and finding the remainder. In this case, when
 is divided by
is divided by
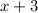 , the remainder is
, the remainder is
 . To determine this, we use the Remainder Theorem, which states that the remainder of a polynomial division can be found by substituting the root of the divisor (in this case,
. To determine this, we use the Remainder Theorem, which states that the remainder of a polynomial division can be found by substituting the root of the divisor (in this case,
 into the polynomial.
into the polynomial.
By substituting
 into the given polynomial, we find that the remainder is
into the given polynomial, we find that the remainder is
 , which simplifies to
, which simplifies to
 , resulting in
, resulting in
 . This remainder can be expressed as
. This remainder can be expressed as
 , which is the correct answer.
, which is the correct answer.
Understanding the Remainder Theorem is crucial in polynomial division as it provides a method to find the remainder without fully carrying out the division. It streamlines the process, making it more efficient to determine the remainder when a polynomial is divided by a linear factor.
In summary, the correct answer is
 as the remainder when
as the remainder when
 is divided by
is divided by
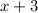 .
.