Question

Determine the extreme values for f(x) = x^4 -8x^2 + 16

Answer 1

The function
`\(f(x) = -x^4 + 16\)` has a local maximum at
`\(x = 0\)` and local minima at
`\(x = -2\) and \(x = 2\)`, with no global maximum or minimum values due to the polynomial's behavior approaching positive infinity as
`\(x\)` goes to positive or negative infinity.

To determine the extreme values of the function f(x)=
`x^4` −
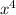`8x^2` +16, we need to find the critical points by setting the derivative equal to zero and then determine the nature of these critical points.

Find the derivative of f(x):

f′ (x)=
`4x^3`−16x

Set the derivative equal to zero and solve for x:

`4x^3` −16x=0

4x(
`x^2`−4)=0

x(x+2)(x−2)=0

So, the critical points are x=0, x=−2, and x=2.

Determine the nature of these critical points using the second derivative test:

f′′ (x)=
`12x^2` −16

For x=0, f′′ (0) =−16 < 0, so x=0 is a local maximum.

For x=−2, f′′ (−2 )= 32 > 0, so x=−2 is a local minimum.

For x=2, f′′ (2) = 32 > 0, so x=2 is a local minimum.

Therefore, the function has local maximum at x=0 and local minima at x=−2 and x=2. However, since f(x) is a polynomial of even degree (4), it approaches positive infinity as x approaches positive or negative infinity. Therefore, there are no global maximum or minimum values, but there are local maximum and minimum values at the critical points mentioned.