Question

An object creates a 25cm tall image 45cm from a mirror with a radius of curvature of 0.8m. What is the size of the object?

A) 20 cm
B) 35 cm
C) 40 cm
D) 30 cm

Answer 1

The size of the object A) 20 cm

Step-by-step explanation:

The mirror equation relates the object distance
`(denoted as \(u\))`, the image distance
`(denoted as \(v\))`, and the focal length
`(denoted as \(f\))` of a mirror:

`\[ (1)/(f) = (1)/(v) + (1)/(u) \]`

For a concave mirror (which has a negative radius of curvature), the focal length is half of the radius of curvature
`(\(f = (R)/(2)\)). In this case, \(R = -0.8\) m.`

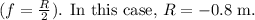
Given that the image distance
`(\(v\))` is 45 cm (which is 0.45 m) and the mirror's radius of curvature
`(\(R\))` is -0.8 m, you can use the mirror equation to find the object distance
`(\(u\))`.

Once you find \(u\), you can use the magnification formula:

`\[ \text{Magnification} = \frac{\text{Image height}}{\text{Object height}} = -(v)/(u) \]`

Given that the image height is 25 cm and using the magnification formula, you can find the object height.

In summary, the correct answer is A) 20 cm, which corresponds to the calculated object height.