Question

For a polynomial function of the fifth degree, determine the polynomial function in the form f(x)=a(x-m)(x-n)(x-p)(x-q)(x-r) using the following graph

Answer 1

Given:

The graph of a polynomial.

To find:

The polynomial function for the given graph.

Solution:

If the graph of function intersect the x-axis at x=c, then (x-c) is a factor of that function f(x).

From the given graph it is clear that the graph of the polynomial function intersect the x-axis at x=-1, x=2, x=3.

So, (x+1), (x-2) and (x-3) are the factors of required polynomial.

At x=2 and x=3, it look like a linear function. So, the multiplicity of (x-2) and (x-3) are 1.

At x=-1, it look like a cubic function. So, the multiplicity of (x+1) is 3.

The required polynomial is

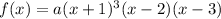`f(x)=a(x+1)^3(x-2)(x-3)` ...(i)

The function passes through the point (1,10). Putting x=1 and f(x)=10, we get

`10=a(1+1)^3(1-2)(1-3)`

`10=a(2)^3(-1)(-2)`

`10=16a`

`(10)/(16)=a`

`0.625=a`

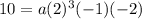
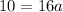
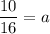
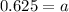
Putting a=0.625 in (i), we get

`f(x)=0.625(x+1)^3(x-2)(x-3)`

`f(x)=0.625(x+1)(x+1)(x+1)(x-2)(x-3)`

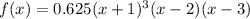
Therefore, the required polynomial function is
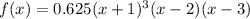`f(x)=0.625(x+1)^3(x-2)(x-3)` or it can be written as
`f(x)=0.625(x+1)(x+1)(x+1)(x-2)(x-3)`.