Question

Insure.com reports that the mean annual premium for automobile insurance in the United States was $1,365 in 2018. Being from Pennsylvania, you believe automobile insurance is cheaper there, and you wish to develop statistical support for your opinion. A sample of 25 automobile insurance policies from the state of Pennsylvania showed a mean annual premium of $1,286 with a standard deviation of s = $165. (Use = 0.05.) Find the P Value

Answer 1

If p<α, you would reject the null hypothesis.If p≥α, you would not reject the null hypothesis.

The general approach is to compare the calculated p-value with the significance level (α):

If p<α, you would reject the null hypothesis.

If p≥α, you would not reject the null hypothesis.

Given data:

- Population mean
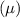`(\(\mu\))`: $1,365

- Sample mean
`(\(\bar{x}\))`: $1,286

- Standard deviation of the sample (s0: $165)

- Sample size (n): 25

- Significance level
`(\(\alpha\))`: 0.05

Null hypothesis
`(\(H_0\))` and alternative hypothesis
`(\(H_1\))`:

`\[ H_0: \mu = \$1,365 \]`

`\[ H_1: \mu < \$1,365 \]`

Calculate the t-value using the formula:

`\[ t = \frac{\bar{x} - \mu}{s/√(n)} \]`

`\[ t = (1286 - 1365)/(165/√(25)) \]`

`\[ t = (-79)/(165/5) \]`

`\[ t \approx -3.7879 \]`

Now, with a t-value of -3.7879 and 24 degrees of freedom (\(n-1\)), you can find the p-value associated with this t-value. Using statistical software or a t-distribution table, you would find the p-value.

The p-value is the probability of obtaining a t-value as extreme as or more extreme than the one observed, assuming the null hypothesis is true. In this case, it's the probability of observing a t-value less than -3.7879.

Once you find the p-value, you can compare it to the significance level
`(\(\alpha = 0.05\))` to make a decision about whether to reject the null hypothesis.

If the p-value is less than 0.05, you would reject the null hypothesis.