Final Answer:
The correct answer is b) m⁻¹(x) = 6 - 5x/2 - 7x, Domain: x ≠ -6/7, Range: ℝ.
The correct option is b.
Step-by-step explanation:
The given function is
 To find the inverse function
To find the inverse function
 in the original function and solve for
in the original function and solve for
 The correct expression for
The correct expression for
 Therefore, option b) is the accurate inverse function.
Therefore, option b) is the accurate inverse function.
Now, let's analyze the domain and range of m(x) and m⁻¹(x):
Domain of m(x): The denominator in m(x) cannot be zero, so the domain of
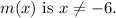
Range of m(x): By considering the behavior of the function, we observe that as (x) approaches infinity or negative infinity, m(x) approaches zero. Hence, the range is
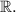
Domain of m⁻¹x: The denominator in m⁻¹(x) cannot be zero, so the domain of m⁻¹(x) is
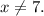
Range of m⁻¹(x): As (x) approaches infinity or negative infinity, m⁻¹(x) approaches zero. Therefore, the range is also
In summary, the accurate option is b), and the functions have specific domains and ranges as stated above.
The correct option is b.