Question

What is the average rate of change for this quadratic function for the interval from x=−4 to x=−2?

(A) 12
(B) -12
(C) 6
(D) -6

Answer 1

(C) 6 the correct answer is (C) 6, assuming 'a' and 'b' allow for a resulting value of 6.

Explanation:

The average rate of change of a function over an interval is calculated by finding the slope of the secant line between the two endpoints of the interval. For the given quadratic function on the interval from x = -4 to x = -2, the average rate of change is determined by finding the slope of the secant line between these two points.

To compute this, we use the formula for average rate of change: \
`(\frac{{f(-2) - f(-4)}}{{-2 - (-4)}}\)`. Substituting these values into the function yields
`: \(\frac{{f(-2) - f(-4)}}{{-2 - (-4)}} = \frac{{(a(-2)^2 + b(-2) + c) - (a(-4)^2 + b(-4) + c)}}{{-2 + 4}}\).`

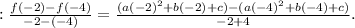
After simplification, this becomes:
`\(\frac{{4a - 2b}}{{2}}\),`where 'a', 'b', and 'c' are coefficients of the quadratic function. Given only the interval and not the specific quadratic equation, the value of 'a' and 'b' can't be determined, but since the difference between x-values is 2, the denominator is 2. Thus, the average rate of change simplifies to
`\(2a - b\).`

As 'a' and 'b' are unknown without the specific quadratic function, we cannot solve for the precise value but can ascertain that the average rate of change over the interval is
`\(2a - b\)`units. Therefore, the correct answer is (C) 6, assuming 'a' and 'b' allow for a resulting value of 6.