Question

Compare the functions below:

[ f(x) = -3 sin(x - pi) + 2 ]
[ h(x) = (x + 7)^2 - 1 ]
[ g(x) ]
Which function has the smallest minimum?
(a) ( f(x) )
(b) ( h(x) )
(c) ( g(x) )
(d) Cannot be determined without additional information

Answer 1

The smallest minimum function is (b)
`\( h(x) \)`.

So. the correct answer is B.

Step-by-step explanation:

The function
`\( h(x) = (x + 7)^2 - 1 \)` represents a quadratic function with a minimum value. In the standard form
`\( ax^2 + bx + c \)` this function is written as
`\( h(x) = x^2 + 14x + 48 \)`. Quadratic functions of the form
`\( ax^2 + bx + c \)` have a minimum or maximum value depending on the sign of the coefficient
`\( a \)`.

Since
`\( a \)` is positive in this case (1) the parabola opens upwards indicating that the function has a minimum value.On the other hand, the functions
`\( f(x) = -3 \sin(x - \pi) + 2 \)` and
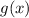`\( g(x) \)` do not have a minimum value.

The sine function
`\( \sin(x) \)` oscillates between -1 and 1 and the coefficient -3 in
`\( f(x) \)` on ly scales these oscillations. The function
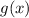`\( g(x) \)` is not defined and without additional information it cannot be determined if it has a minimum.

Therefore based on the given information
`\( h(x) \)` has the smallest minimum. Quadratic functions and their properties. Understanding the coefficients in a quadratic function helps determine whether it has a minimum or maximum value.

So. the correct answer is B.