Question

What is the distance (in units) between points C and D? Round your answer to the nearest hundredth.

Answer 1

Given:

The two points on a coordinate plane are C(-5,-1) and D(0,3).

To find:

The distance between C and D.

Solution:

Distance formula:

`d=√((x_2-x_1)^2+(y_2-y_1)^2)`

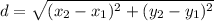
Using the distance formula, the distance between C(-5,-1) and D(0,3) is

`CD=√((0-(-5))^2+(3-(-1))^2)`

`CD=√((0+5)^2+(3+1)^2)`

`CD=√((5)^2+(4)^2)`

`CD=√(25+16)`

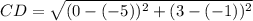
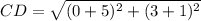
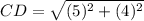
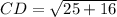
On further simplification, we get

`CD=√(41)`

`CD=6.40312424`

`CD\approx 6.40`

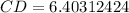
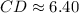
Therefore, the distance between C and D is 6.40 units.