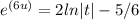The solution to the differential equation with the given initial condition is:
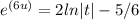
To solve the separable differential equation: u
 , we can rearrange the equation as follows:
, we can rearrange the equation as follows:
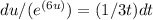
Now, we can integrate both sides.
On the left side, we can use the substitution v = 6u, which means dv = 6 du:
(1/6) ∫
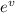 dv = (1/3) ∫ (1/t) dt
dv = (1/3) ∫ (1/t) dt
Integrating both sides, we get:
(1/6)
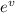 = (1/3) ln|t| + C
= (1/3) ln|t| + C
Now, substitute back v = 6u:
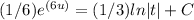
Finally, to find the particular solution, we can use the given initial condition u(0) = -5:
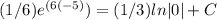
Simplifying, we find C = -5/6.
Therefore, the solution to the differential equation with the given initial condition is: