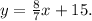The equation in slope-intercept form is
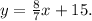
Let's determine the equation in slope-intercept form step by step.
We want to find the equation in the form
 , where m is the slope and b is the y-intercept.
, where m is the slope and b is the y-intercept.
Step 1: Calculate the Slope (\(m\))
![\[ m = \frac{\text{Change in } Y}{\text{Change in } X} \]\[ m = (36 - 12)/(18 - (-3)) \]\[ m = (24)/(21) \]\[ m = (8)/(7) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/ayj1caouxlthghr00hbnu51p16vcruf2wq.png)
So, the slope m is
 .
.
Step 2: Use the Slope-Intercept Form \(y = mx + b\) and substitute the slope:
![\[ y = (8)/(7)x + b \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/tt64pziyizi5gu5gliaz38iiyjuhomft5y.png)
Now, we need to find the y-intercept (\(b\)). We can use one of the given points (let's use (-3, 12)) to solve for \(b\).
![\[ 12 = (8)/(7) \cdot (-3) + b \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/u16k9qgbrn91yzr93lsb93wiebor3v4id7.png)
Now, solve for b:
![\[ 12 = -(24)/(7) + b \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/or9i9i0pxetwnbk8dq9o6hadyl92g83wov.png)
To simplify, multiply both sides by 7 to get rid of the fraction:
![\[ 84 = -24 + 7b \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/pnrn60elcpv12ej9wxbu0mdskp7dlhljuc.png)
Add 24 to both sides:
![\[ 7b = 108 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/tw9u32n8b8xqzvo4t5rkf34lk1oaytqkzm.png)
Divide by 7:
![\[ b = 15 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/1hw19i9gvtk6vawxva4vdoaqbld5gzo0tp.png)
So, the y-intercept b is 15.
Step 3: Substitute the slope and y-intercept back into the equation:
![\[ y = (8)/(7)x + 15 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/gt4x71p07zrco3gb1od5btrukb146fikxh.png)
Therefore, the equation in slope-intercept form is