Final Answer:
we know the asymptotic behavior for the kinetic energy
![(\(T_s[\rho]\))](https://img.qammunity.org/2024/formulas/physics/high-school/5z592oxbl2cri4bqojkvn8f6mcm4bc7hpo.png) and total energy
and total energy
![(\(E[\rho]\))](https://img.qammunity.org/2024/formulas/physics/high-school/q6q9ukfgz0kyf5fz6b6ldd3epf0hi37ldz.png) in Density Functional Theory (DFT) is known.
in Density Functional Theory (DFT) is known.
Step-by-step explanation:
In the asymptotic behavior of the kinetic energy,
![\(T_s[\rho]\)](https://img.qammunity.org/2024/formulas/physics/high-school/xnj98qudwgi0k0qcr4xxhk4pf0wis6zsty.png) , the dependence on the electron density
, the dependence on the electron density
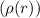 as r goes to infinity is characterized by the term
as r goes to infinity is characterized by the term
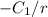 . This term reflects the long-range behavior of the kinetic energy density in the presence of a finite number of electrons. The constant
. This term reflects the long-range behavior of the kinetic energy density in the presence of a finite number of electrons. The constant
 captures the system-specific details, and the negative sign signifies the decrease in kinetic energy as electrons move away.
captures the system-specific details, and the negative sign signifies the decrease in kinetic energy as electrons move away.
Similarly, for the total energy,
![\(E[\rho]\)](https://img.qammunity.org/2024/formulas/physics/high-school/i94oazqlkhhrjlso6n9h2mruvy2d68viql.png) , the asymptotic behavior is also characterized by a term that goes as
, the asymptotic behavior is also characterized by a term that goes as
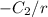 as r approaches infinity. Here,
as r approaches infinity. Here,
 represents another constant that encapsulates the system's characteristics. The negative sign indicates the decrease in total energy as one moves to the outer regions of the system. Understanding these asymptotic behaviors is crucial in analyzing the long-range effects in DFT calculations, providing insights into the behavior of the system at large distances.
represents another constant that encapsulates the system's characteristics. The negative sign indicates the decrease in total energy as one moves to the outer regions of the system. Understanding these asymptotic behaviors is crucial in analyzing the long-range effects in DFT calculations, providing insights into the behavior of the system at large distances.
In summary, the asymptotic behaviors of the kinetic and total energies in DFT involve terms proportional to
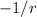 , where constants
, where constants
 and
and
 encapsulate the system-specific details, offering valuable information about the long-range behavior of these energy components.
encapsulate the system-specific details, offering valuable information about the long-range behavior of these energy components.