Question

Find the value of x, round your answer to the nearest tenth.

Answer 1

`\boxed {\boxed {\sf x \approx 3.7}}`

Explanation:

Since we are given a right triangle, we can use the trigonometric ratios.

• sin(θ)=opposite/hypotenuse
• cos(θ)= adjacent/hypotenuse
• tan(θ)=opposite/adjacent

We have the angle 24 and the sides 9 and x. 9 is the hypotenuse because it's opposite the right angle. x is the opposite, because it as across from the 24 degree angle. So, we have:

• hypotenuse= 9
• opposite=x

Therefore, we must use sine.

`sin(24)= (x)/(9)`

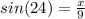
We are trying to solve for the value of x, so we must isolate the variable. x is being divided by 9. The inverse of division is multiplication. Multiply both sides by 9.

`9*sin(24)= (x)/(9)*9`

`9*sin(24)=x`

`9*0.406736643=x`

`3.66062979=x`

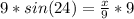
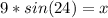
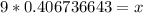
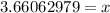
Round to the nearest tenth. The 6 in the hundredth place tells us to round the 6 to a 7.

`3.7 \approx x`

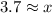
x is approximately 3.7