Question

Minimize the objective function P = 45x + 48y for the given constraints.

X20

y 20

5x + y = 16

x + 3y 26

Answer 1

Following are the solution to the given equation:

Explanation:

The graph file and correct question are defined in the attachment please find it.

According to the linear programming principle, we predict, that towards the intersections of the constraint points in the viability area, and its optimal solution exists. The sketch shows the points that are (0,16), (3,1), and (6,0).

by putting each point value into the objective function:

`\to P(0,16) = 768\\\\\to P(3,1) = 183\\\\\to P(6,0) = 270`

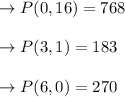
Thus, the objective of the function is reduced with a value of 183 at (3,1).