There is one real and repeated solution to the equation f(x)=0. There are one real and equal solutions. The equation for the function is
 . The solutions are
. The solutions are
 and
and
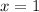 .
.
The graph of the quadratic function is a declining curve. The equation f(x) = 0 represents the values of x for which the function equals zero.
Type of solutions: Since the graph is a quadratic curve that touches the x-axis at a single point (the vertex), the solutions are real and equal.
Number of solutions: As the line intersects the x-intercept once, there is one real and equal solution.
The equation for the Function:
Given that the vertex of the graph is at (2, 2), we can use the vertex form of a quadratic equation, which is
 , where
, where
 is the vertex of the parabola.
is the vertex of the parabola.
Plug in the values:
![\[ f(x) = a(x - 2)^2 + 2 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/wednt5ucqvjp9iofum5awtfwyuy2exdxj3.png)
To find the value of a, use one of the points on the graph, for example, (0, -6):
![\[ -6 = a(0 - 2)^2 + 2 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/11sbkypxuej68ixz0u3571z71pz0dco0kt.png)
Solve for a:
![\[ -6 = 4a + 2 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/p90a8yvmsuinnt9fn3mdp6a955mabjyj8z.png)
![\[ 4a = -8 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/vw9ajsq7yl6nuxe5g09tbad8119tczkvdd.png)
![\[ a = -2 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/c7j00ifqfd6hnxd6w6fchwye5i79pgpecu.png)
So, the equation for the function is
 .
.
Solution(s) of the Equation
 :
:
Set f(x) equal to 0 and solve for x:
![\[ -2(x - 2)^2 + 2 = 0 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/oyf0umkm3t7cwhxgrv96uuufk7gxauw5ij.png)
![\[ -2(x - 2)^2 = -2 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/q4w387s9ncto8s75zra1ax83nx18kfjk0c.png)
![\[ (x - 2)^2 = 1 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/xiqwdta8xnzcu3npdgrqp9y2924arvcnn5.png)
![\[ x - 2 = \pm 1 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/dnmguq3srqaz03803l7sl01fcxps94xezh.png)
![\[ x = 3 \text{ or } x = 1 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/c6iizc90itqf2ulfoz6f7cb0db3az6zrev.png)
So, the solutions are
 and
and
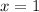 .
.