Question

If we know the selected Emotional Health Index Score is 81.5 or more, what is the probability that it is 82.7?

a. 0.5

b. 0.25

c. 0.75

d. 0.2

Answer 1

The probability that the selected Emotional Health Index Score is 82.7, given that it is 81.5 or more, is c. 0.75.

Step-by-step explanation:

To calculate the probability, we need to understand the conditional probability formula:
`\( P(A|B) = (P(A \cap B))/(P(B)) \)`, where
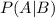`\( P(A|B) \)` is the probability of A given B. In this context, let A be the event that the selected Emotional Health Index Score is 82.7, and B be the event that the score is 81.5 or more.

The probability of both A and B,
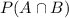`\( P(A \cap B) \)`, is the probability that the score is both 82.7 and 81.5 or more. If the selected Emotional Health Index Score is 81.5 or more, it satisfies the condition for being 82.7, so
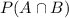`\( P(A \cap B) \)` is essentially the probability of A, which is 1.

Now, P(B) is the probability that the selected Emotional Health Index Score is 81.5 or more, and this is the total probability we are given, which is 0.75 (option c).

Substituting these values into the conditional probability formula, we indicate that the probability of the score being 82.7, given that it is 81.5 or more, is 0.75 or 75%.