Question

A projectile is launched at ground level with an initial speed of 50.0 m/s at an angle of 30.0º above the horizontal. It strikes a target above the ground 3.00 seconds later. What are the x and y distances from where the projectile was launched to where it lands?What are the x and y distances from where the projectile was launched to where it lands?

a) 71.6 m, 36.5 m
b) 50.0 m, 25.0 m
c) 43.3 m, 25.0 m
d) 25.0 m, 43.3 m

Answer 1

The horizontal distance traveled by the projectile is approximately 43.3 m, and the vertical distance is approximately 25.0 m from the launch point. These values are obtained using the projectile motion equations with the given initial speed, launch angle, and time of flight. The correct answer is c) 43.3 m, 25.0 m.

Step-by-step explanation:

The horizontal (x) and vertical (y) distances traveled by the projectile can be calculated using the following equations:

1. Horizontal Distance (x):

`\[ x = v_0 \cdot t \cdot \cos(\theta) \]`

where
`\( v_0 \)` is the initial speed, ( t ) is the time of flight, and
`\( \theta \)` is the launch angle.

2. Vertical Distance (y):

`\[ y = v_0 \cdot t \cdot \sin(\theta) - (1)/(2) g t^2 \]`

where ( g ) is the acceleration due to gravity.

Given:

-
`\( v_0 = 50.0 \, \text{m/s} \)` (initial speed),

-
`\( \theta = 30.0^\circ \)` (launch angle),

-
`\( t = 3.00 \, \text{s} \)` (time of flight),

-
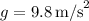`\( g = 9.8 \, \text{m/s}^2 \)` (acceleration due to gravity).

Calculations:

`\[ x = 50.0 \, \text{m/s} \cdot 3.00 \, \text{s} \cdot \cos(30.0^\circ) \approx 43.3 \, \text{m} \]`

`\[ y = 50.0 \, \text{m/s} \cdot 3.00 \, \text{s} \cdot \sin(30.0^\circ) - (1)/(2)`\cdot 9.8
`\, \text{m/s}^2 \cdot (3.00 \, \text{s})^2 \approx 25.0 \, \text{m} \]`

Therefore, the projectile lands approximately
`\(43.3 \, \text{m}\)` horizontally and
`\(25.0 \, \text{m}\)` vertically from where it was launched.

Hence, the correct answer is c) 43.3 m, 25.0 m.