To find the concentration of
 when
when
 starts to precipitate in a 0.0125 M Ag+ solution, we use the Ksp value for
starts to precipitate in a 0.0125 M Ag+ solution, we use the Ksp value for
 . The Ksp expression for
. The Ksp expression for
 is Ksp =
is Ksp =
![[Ag+]^3[(PO_4)^( 3-)]](https://img.qammunity.org/2024/formulas/chemistry/high-school/cfu6n8unjpn74tcev8ewtbzaun43buyzr6.png) . With the Ksp value and given Ag+ concentration, we can solve for [
. With the Ksp value and given Ag+ concentration, we can solve for [
 3-].
3-].
To calculate the concentration of
 when
when
 starts to precipitate from a solution that is 0.0125 M in Ag+, we need to use the solubility product constant (Ksp) for
starts to precipitate from a solution that is 0.0125 M in Ag+, we need to use the solubility product constant (Ksp) for
 .
.
The chemical equation for the dissolution of
 is:
is:
 (s) ⇌ 3Ag+(aq) +
(s) ⇌ 3Ag+(aq) +
 (aq)
(aq)
The Ksp expression is:
Ksp =
![[Ag+]^3[(PO_4)^( 3-)]](https://img.qammunity.org/2024/formulas/chemistry/high-school/cfu6n8unjpn74tcev8ewtbzaun43buyzr6.png)
Given the Ksp for
 , we can plug in the concentration of Ag+ and solve for the concentration of
, we can plug in the concentration of Ag+ and solve for the concentration of
 .
.
Let's assume the Ksp for
 is 'x'. The concentration of Ag+ is already given as 0.0125 M. Since there are 3 moles of Ag+ for each mole of
is 'x'. The concentration of Ag+ is already given as 0.0125 M. Since there are 3 moles of Ag+ for each mole of
 that dissolves, the concentration of Ag+ ions will be three times the concentration of
that dissolves, the concentration of Ag+ ions will be three times the concentration of
 ions. Therefore, we set up the equation as follows:
ions. Therefore, we set up the equation as follows:
Ksp =
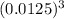 [
[
 ]
]
x =
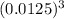 [
[
 ]
]
To find the concentration of
 , we divide both sides by
, we divide both sides by
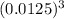 :
:
[
 ] = x/
] = x/
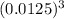
Without the actual Ksp value, we cannot provide the exact concentration, but this is the process that would be used to calculate it. Once Ksp is known, we can plug it in and solve for [
 ].
].
If we assume the Ksp for
 is a known value, it's possible to directly calculate [
is a known value, it's possible to directly calculate [
 ], but since it hasn't been provided, we cannot complete the calculation.
], but since it hasn't been provided, we cannot complete the calculation.