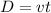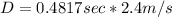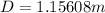Question

Steps/Formulas Please!

A ball is launched up a semicircular chute with radius 0.57 m in such a way that at the top of the chute, just before it leaves, the ball has a radial acceleration of magnitude 12.4 m/s^2.


How far from the bottom of the chute does the ball land?

Answer 1

`D=1.15608m`

Step-by-step explanation:

From the question we are told that

Radius of chute
`r=0.57`

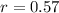
Magnitude of radial acceleration
`a_r=12.4m/s^2`

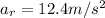
Generally the equation for the centripetal acceleration is mathematically given by

`a_r=(v^2)/(R)`

`v=√(0.57*12.4)`

`v=2.4m/s`

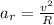
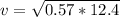
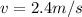
Generally the equation for the motion is mathematically given by

`S= ut +0.5at^2`

`t^2= (S-ut)/(1/2a)`

`t^2= ((2*0.57)-0)/(1/2*9.8)`

`t^2= 0.232`

`t= √( 0.232)`

`t= 0.4817sec`

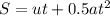
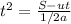
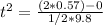
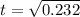
Generally the equation for the distance traveled is mathematically given by

`D=vt`

`D=0.4817sec*2.4m/s`

`D=1.15608m`