Final Answer:
The coefficient of kinetic friction between the block and the surface is 0.35. Thus the correct answer is option c) 0.35.
Step-by-step explanation:
The coefficient of kinetic friction (μ_k) can be determined by considering the energy transformations in the system. When the block is pushed against the spring, potential energy stored in the spring is given by the formula:
 ,
,
where k is the force constant (spring constant) and x is the displacement from equilibrium. In this case,
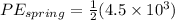
 .
.
This potential energy is converted into kinetic energy as the block is released and slides. The kinetic energy (KE) of the block can be expressed as:
 , where m is the mass of the block and v is its velocity.
, where m is the mass of the block and v is its velocity.
The work done by friction
 during the sliding is given by
during the sliding is given by
 , where d is the displacement and g is the acceleration due to gravity.
, where d is the displacement and g is the acceleration due to gravity.
The work done by friction is equal to the change in mechanical energy
 .
.
By substituting the relevant formulas, the equation becomes
 .
.
Solving for
 we get
we get
 , where s is the distance over which friction acts.
, where s is the distance over which friction acts.
Substituting the given values,
 , which yields
, which yields
 .
.
Therefore, the coefficient of kinetic friction between the block and the surface is approximately 0.35.
Thus the correct answer is option c) 0.35.