Answer:
ω = 5.5 rad/s
Step-by-step explanation:
- Assuming no external torques present during the instant that the clump of clay is dropped on the turntable, total angular momentum must be conserved.
- The angular momentum of a rotating rigid body, can be expressed as follows:
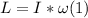
where I = moment of inertia regarding the axis of rotation, and ω =
angular speed of the rotating body.
- Since the angular momentum must keep constant, this means that it must be satisfied the following equality:
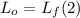
where L₀ = I₀ * ω₀, Lf = If * ωf.
I₀ is the moment of inertia of a solid disk rotating around an axis
passing through its center, as follows:

If, is the moment of inertia after dropping the clump of clay, which adds
its own moment of inertia as a point mass, as follows:
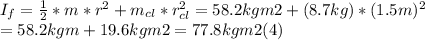
- Replacing I₀, If and ω₀ in (2), we can solve for ωf, as follows:
