Answer:
Vertex:
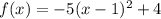
Standard:

Factored:
This is unfactorable.
Explanation:
The parabola has a vertex at (1, 4) and it crosses a point at (2, -1).
We will start off with the vertex form, given by:
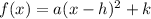
Where (h, k) is the vertex.
Therefore:
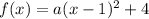
Since the function passes through (2, -1), f(x) = -1 when x = 2:

Solve for a:
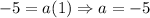
Therefore, vertex form is:
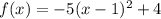
To find the standard form, expand:

Distribute:
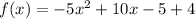
And simplify:

We can now factor. Which two values multiply to be 5 and add up to be 10?
Since this is no possible, the equation is unfactorable.