Therefore, the value of the exterior angle of the triangle is
.
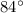The exterior angle theorem states that the measure of the exterior angle of a triangle is equal to the sum of two remote interior angles. Here the exterior angle is
and the two remote interior angles are
and
. Hence,
2x+18+24=3x+16
3x-2x=18+24-16
x=26
Substituting the value of x in (3x+16), we get:
3(26)+6=78+6
=
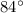
Therefore, the value of the exterior angle of the triangle is
.
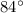