Question

Select the correct answer.

What is the range of function g?
g(x)
-6 -4 -2
6
4
2-
9
-6
O
46
X

Answer 1

The range of the function g(x) = 6 - 2x + x^2 given the domain
`\( D = \{-1, 0, 1, 2\} \)` is
`\( \{9, 6, 5\} \)`.



To find the range of the function
`\( g(x) = 6 - 2x + x^2 \)` given the domain
`\( D = \{-1, 0, 1, 2\} \)`, we can follow these steps:

1. **Find the values of
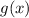`\( g(x) \)` for each
`\( x \)` in the given domain:**

- For x = -1:

[ g(-1) = 6 - 2(-1) + (-1)^2]

[ g(-1) = 6 + 2 + 1 = 9]

- For x = 0:

[g(0) = 6 - 2(0) + (0)^2]

[ g(0) = 6]

- For x = 1:

[g(1) = 6 - 2(1) + (1)^2]

[g(1) = 6 - 2 + 1 = 5 ]

- For x = 2:

[ g(2) = 6 - 2(2) + (2)^2]

[ g(2) = 6 - 4 + 4 = 6]

2. **Determine the range by considering the values of
`\( g(x) \)`:**

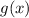- The values of g(x) for the given domain are
`\( \{9, 6, 5, 6\} \)`.

3. **Identify the range:**

- The range is the set of all unique
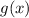`\( g(x) \)` values. In this case, the range is
`\( \{9, 6, 5\} \)`.

So, the range of the function g(x) = 6 - 2x + x^2 given the domain
`\( D = \{-1, 0, 1, 2\} \)` is
`\( \{9, 6, 5\} \)`.



The probable question can be: What is the range of the function g(x) = 6 - 2x + x2 given the domain D = {-1, 0, 1, 2}?