Question

Which function models the mass of the substance in grams, R(t), after decaying for t years?

R(t) = (100)^t
R(t) = 100-t1/2
R(t) = 100-1/2t
R(t) = 100()^2

Answer 1

The correct option is the first one:
`\( R(t) = (100)^t \)`

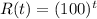
The function that typically models the decay of a substance over time is an exponential decay function.

The correct expression for modeling the mass of a substance in grams after decaying for
`\( t \)` years is typically given by the exponential decay formula:

`\[ R(t) = R_0 \cdot e^(-kt) \]`

Here:

-
`\( R(t) \)` is the mass of the substance at time
`\( t \)`,

-
`\( R_0 \)` is the initial mass of the substance,

-
`\( e \)` is the mathematical constant approximately equal to 2.71828,

-
`\( k \)` is the decay constant.

The correct choice from the options you provided is:

`\[ R(t) = (100)^t \]`

This is an exponential function where the base is less than 1 (in this case,
`\( (1)/(100) \)`), indicating decay.

The exponent t represents the time in years, and R(t) gives the mass of the substance at time t. The base raised to the power of t ensures that the mass decreases over time.

So, the correct option is the first one:
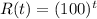`\( R(t) = (100)^t \)`.