Question

A cylindrical bar of metal having a diameter of 20.3 mm and a length of 205 mm is deformed elastically in tension with a force of 46400 N. Given that the elastic modulus and Poisson's ratio of the metal are 66.9 GPa and 0.32, respectively, determine the following: (a) The amount by which this specimen will elongate in the direction of the applied stress. (b) The change in diameter of the specimen. Indicate an increase in diameter with a positive number and a decrease with a negative number.

Answer 1

a) 0.4393 mm

b) -0.141 mm

Step-by-step explanation:

Cylindrical bar : Diameter = 20.3 mm , Length = 205 mm

Force that deforms bar of metal = 46400 N

elastic modulus = 66.9 GPa

Poisson's ratio ( u ) = 0.32

A) Determine the amount by which this specimen will elongate in the direction of applied stress

dl =
`(P*L)/(A*E)`

where P = 46400 N

L = 205 mm

A =
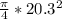`(\pi )/(4) * 20.3^2` = 323.65

E = 66.9 GPa

dl = ( 46400 * 205 ) / ( 323.65 * 66.9 * 10^3 ) = 0.4393 mm

B) determine the change in diameter of the specimen

change in diameter( compressed due to elongation in length )

= - u * dl

= - 0.32 * 0.4393

= -0.141 mm