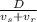Question

The next four questions refer to the situation below.

A person is swimming in a river with a current that has speed vR with respect to the shore. The swimmer first swims downstream (i.e. in the direction of the current) at a constant speed, vS , with respect to the water. The swimmer travels a distance D in a time tOut . The swimmer then changes direction to swim upstream (i.e. against the direction of the current) at a constant speed, vS , with respect to the water and returns to her original starting point (located a distance D from her turn-around point) in a time tIn .
What is tOut in terms of vR, vS, and D, as needed?

Answer 1

t_{out} =
`(v_s - v_r)/(v_s+v_r)` t_{in}, t_{out} =
`(D)/(v_s +v_r)`

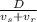
Step-by-step explanation:

This in a relative velocity exercise in one dimension,

let's start with the swimmer going downstream

its speed is

`v_(sg 1) = v_(sr) + v_(rg)`

The subscripts are s for the swimmer, r for the river and g for the Earth

with the velocity constant we can use the relations of uniform motion

`v_(sg1)` = D /
`t_(out)`

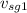D = v_{sg1} t_{out}

now let's analyze when the swimmer turns around and returns to the starting point

`v_(sg 2) = v_(sr) - v_(rg)`

`v_(sg 2)` = D /
`t_(in)`

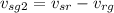
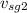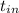
D = v_{sg 2} t_{in}

with the distance is the same we can equalize

`v_(sg1) t_(out) = v_(sg2) t_(in)`

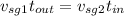
t_{out} = t_{in}

t_{out} =
`(v_s - v_r)/(v_s+v_r)` t_{in}

This must be the answer since the return time is known. If you want to delete this time

t_{in}= D /
`v_(sg2)`

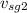
we substitute

t_{out} = \frac{v_s - v_r}{v_s+v_r} ()

t_{out} =
`(D)/(v_s +v_r)`