Question

You have a data set with a Gas Price variable. It lists the price of a gallon of regular gasoline at a randomly selected 100 gas stations around the country in a particular week. You calculate a 95% confidence interval for the mean of Gas Price, and it turns out to be $2.43 plus or minus $0.16. Which of the following most closely reflects what you can conclude? a. If your sample size had been 400, and you had observed the same sample mean but a sample standard deviation twice as large as in your original sample, the 95% confidence interval would have been approximately $2.43 plus or minus $0.08. b. If your sample size had been 400 and you had obtained the same sample mean and sample standard deviation as in your original sample, the 95% confidence interval would have been approximately $2.43 plus or minus $0.08. c. If you had observed the same sample mean and sample size, but a sample standard deviation twice as large as the one you observed, the 95% confidence interval would have been approximately $2.43 plus or minus $0.24. d. If you had observed the same sample mean and sample size, but a sample standard deviation half as large as the one you observed, the 95% confidence interval would have been approximately $2.43 plus or minus $0.04.

Answer 1

b. If your sample size had been 400 and you had obtained the same sample mean and sample standard deviation as in your original sample, the 95% confidence interval would have been approximately $2.43 plus or minus $0.08.

Explanation:

Confidence interval:

A confidence interval is given by the sample mean plus minus the margin of error.

Margin of error:

`M = z(\sigma)/(√(n))`

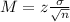
In which M is the margin of error, z is related to the confidence level(the higher the confidence level, the higher the value of Z),
`\sigma` is the standard deviation and n is the size of the sample.

This is important to note that the margin of error is inversely proportional to the square root of the size of the sample.

In this question, we have that:

95% confidence interval for the mean of Gas Price is of $2.43 plus or minus $0.16, with a sample of 100.

Option A:

Sample size of 400, which is four times the original, while the standard deviation is twice the original.

This means that the margin of error would be the same, as it would be multiplied by 2 in the numerator and divided by the square root of 4, that is, 2 in the denominator. So the interval would still be of $2.43 plus or minus $0.16, which means that option a. is wrong.

Option B:

Sample size of 400, which is four times the original, while the rest is the same.

The margin of error would be divided by the square root of 4, that is, 2, which means that it would be half of the original, and the interval would be $2.43 plus or minus $0.08, which means that option b. is correct.

Option C:

In this case, the margin of error will be doubled, since the standard deviation is double the original. So the confidence interval would be of $2.43 plus or minus $0.32, which means that option c. is wrong.

Option D:

In this case, the margin of error will be halved, since the standard deviation is half the original. So the confidence interval would be of $2.43 plus or minus $0.08, which means that option d. is wrong.