Question

Solve using Law of exponents, Zero Exponent, and Negative Exponent​

Answer 1

`2 {}^( - 15) {a}^(3) b {}^(15) c {}^(6) {d}^( - 27)`

Answer 2

`(a^(3) b^(15)c^(6))/(2^(15)d^(27))`

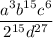
Explanation:

Given expression:

`\left[(2^(-3)ab^0(c^(-2)d^3)^(-2))/(2^2a^0(b^5c^(-2))^(-1)d^3) \right]^3`

Following the order of operations, begin by applying exponent rules to the parentheses in the numerator and denominator.

`\textsf{Apply exponent rule} \quad (a^b)^c=a^(bc):`

`\implies \left[(2^(-3)ab^0c^((-2 \cdot -2))d^((3 \cdot -2)))/(2^2a^0b^((5 \cdot -1))c^((-2 \cdot -1))d^3) \right]^3`

`\implies \left[(2^(-3)ab^0c^(4)d^(-6))/(2^2a^0b^(-5)c^(2)d^3) \right]^3`

Notice there are two terms with a zero exponent.

`\textsf{Apply exponent rule} \quad a^0=1:`

`\implies \left[(2^(-3)a(1)c^(4)d^(-6))/(2^2(1)b^(-5)c^(2)d^3) \right]^3`

`\implies \left[(2^(-3)ac^(4)d^(-6))/(2^2b^(-5)c^(2)d^3) \right]^3`

Separate the terms:

`\implies \left[(2^(-3))/(2^2) \cdot (a)/(b^(-5)) \cdot (c^(4))/(c^(2)) \cdot (d^(-6))/(d^3) \right]^3`

`\textsf{Apply exponent rule} \quad (a^b)/(a^c)=a^(b-c):`

`\implies \left[2^((-3-2)) \cdot (a)/(b^(-5)) \cdot c^((4-2)) \cdot d^((-6-3))\right]^3`

`\implies \left[2^(-5) \cdot (a)/(b^(-5)) \cdot c^(2) \cdot d^(-9)\right]^3`

`\textsf{Apply exponent rule} \quad (1)/(a^(-n))=a^n:`

`\implies \left[2^(-5) \cdot a \cdot b^(5) \cdot c^(2) \cdot d^(-9)\right]^3`

`\textsf{Apply exponent rule} \quad (a^b)^c=a^(bc):`

`\implies 2^((-5 \cdot 3)) \cdot a^((1\cdot 3)) \cdot b^((5\cdot 3)) \cdot c^((2\cdot 3)) \cdot d^((-9\cdot 3))`

`\implies 2^(-15) \cdot a^(3) \cdot b^(15) \cdot c^(6) \cdot d^(-27)`

`\implies 2^(-15) a^(3) b^(15) c^(6) d^(-27)`

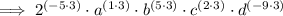
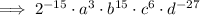
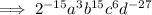
To give the expression as a rational with positive exponents,

`\textsf{apply exponent rule} \quad a^(-n)=(1)/(a^(n)):`

`\implies (1)/(2^(15)) \cdot a^(3) \cdot b^(15) \cdot c^(6) \cdot (1)/(d^(27))`

`\implies (a^(3) b^(15)c^(6))/(2^(15)d^(27))`

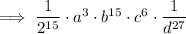
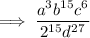
Note: I have left 2¹⁵ as an exponent with base 2. As 2¹⁵ = 32768, you can substitute 2¹⁵ for 32768 in the final answer, if you so wish.