Question

Solve the triangle. Round all answers to two decimal places. 12 17 B 10 a b sin (A) = sin(B) = sin(C) a² = 6² + c² - 2bc cos(A)​

Answer 1

To solve the triangle using the given information:

Given:

Side a = 10

Side b = 12

Side c = 17

We're asked to find angle A and side b.

We'll use the Law of Cosines to find angle A first:

`\[a^2 = b^2 + c^2 - 2bc \cdot \cos(A)\]`

Substitute the given values:

`\[10^2 = 12^2 + 17^2 - 2 * 12 * 17 \cdot \cos(A)\]\[100 = 144 + 289 - 408 \cdot \cos(A)\]\[100 = 433 - 408 \cdot \cos(A)\]`

Solving for \(\cos(A)\):

`\[-333 = -408 \cdot \cos(A)\]\[\cos(A) = (-333)/(-408)\]\[\cos(A) \approx 0.81618\]`

Now, to find angle A, take the arccosine of 0.81618:

`\(A = \arccos(0.81618)\)\(A \approx 36.87^\circ\)`

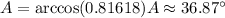
Now that we have angle A, let's use the Law of Sines to find angle B:

`\[(\sin(A))/(a) = (\sin(B))/(b)\]\[(\sin(36.87^\circ))/(10) = (\sin(B))/(12)\]`

Solving for
`\(\sin(B)\):`

`\[\sin(B) = (12 * \sin(36.87^\circ))/(10)\]\[\sin(B) \approx 0.7017\]`

Now, find angle B:

`\(B = \arcsin(0.7017)\)\(B \approx 44.07^\circ\)`

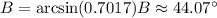
Therefore, in the triangle:

Angle A ≈ 36.87°

Angle B ≈ 44.07°

Explanation: