Question

Find the balance in the account after the given period.

$4000 principal earning 3% compounded annually, 4 years

Answer 1

balance after 4 years = $4502

Explanation:

Formula to get the final amount when compounded annually is,

Final amount =
`\text{Principal amount}* (1+(r)/(n))^(nt)`

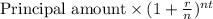
Here, r = rate of interest

n = number of compounding per year

t = Duration of investment

From the given question,

Final amount =
`4000(1+(0.03)/(1))^((1* 4))`

=
`4000(1.03)^4`

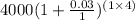
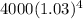
= 4502.0352

≈ $4502

Therefore, balance after 4 years = $4502