Question

If the midpoint of AB is (3,-4).If the coordinates of A are(1,-7),what are the coordinates of B?

Answer 1

(5, -1)

Explanation:

The midpoint is given by the equation:

`\text (x_m, y_m) =((x_1+x_2)/(2),(y_1+y_2)/(2))`, where the coordinates of one endpoint are (x₁, y₁) and the coordinates of the other endpoint are (x₂, y₂).

Determining the Givens

We are given that the two endpoints are A and B. The coordinates of A are (1, -7), and the coordinates of the midpoint is (3, -4).

Substituting these values into the equation, we get:

`(3, -4) = ((1+x_2)/(2),(-7+y_2)/(2))`

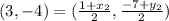
We can split this into two equations, one for the x-coordinate and one for the y-coordinate.

`3 = (1+x_2)/(2)\\-4 = (-7+y_2)/(2)`

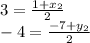
Solve the Equations

First, start by multiplying both sides by 2:

`6 = 1+x_2\\-8 = -7+y_2`

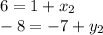
For the first equation, subtract both sides by 1. For the second equation, add 7 to both sides.

`x_2 = 5\\y_2 = -1`

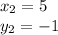
Therefore the coordinates of B are (x₂, y₂) = (5, -1).