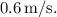Question

The velocity of a vibrating object changes as a function of time as v=−(0.6m/s)cos(2πt). What is the amplitude of the vibration?

a) 0.6 m/s
b) 0.6
c) 2π
d) Not specified

Answer 1

The amplitude of the vibration is 0.6 m/s (Option a).

Step-by-step explanation:

The given expression for velocity is
`\(v = -(0.6 \, \text{m/s}) \cos(2\pi t)\),`where (t) is time. In this expression, the coefficient of the cosine term, which is \
`(0.6 \, \text{m/s}\),` represents the amplitude of the vibration. The negative sign indicates that the vibration is in the opposite direction to the cosine function.

To understand this, let's break down the expression. The general form of a sinusoidal function is
`\(y = A \cos(Bx + C)\)`, where (A) is the amplitude. In our case, (A) is
`\(0.6 \, \text{m/s}\),` confirming that the amplitude is indeed
`\(0.6 \, \text{m/s}\).`

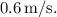
The negative sign signifies that the oscillation is inverted or occurs in the opposite direction along the y-axis. However, since we are interested in the amplitude, which is a scalar quantity, the negative sign doesn't affect our determination of the amplitude. Therefore, the amplitude of the vibration is
`\(0.6 \, \text{m/s}\).`